How to Solve Sudoku Puzzles – A Complete Walkthrough, Part 4

In the figure shown below, there are a few opportunities to quickly fill in some empty spaces. In the column (through the center spaces of the right-hand squares, outlined in green), there is only one empty space remaining. Just by checking through the existing numbers in this column, we know that this one remaining number should be 2. So we can place the number 2 in that spot (marked in blue).
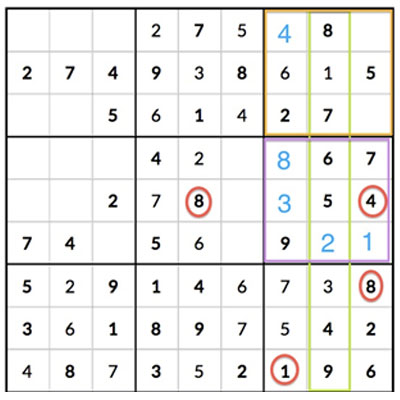
Now that the number 2 is in place, let’s see what else we can do with the rest of the empty spaces in the same square (outlined in purple). We know that there are only three empty spaces remaining, which must contain 1, 3 and 8. If we look to the squares to the left and below, we can see two 8s and a 1 (circled in red) which show us that the 8 must go in the upper left corner of the purple square, and the 1 must go in the lower-right corner of the purple square. That leaves 3 as the only possible number to go in the one remaining space.
Now let’s look to the upper left square of the grid, outlined in orange. We know that this square only has three empty spaces left, and they must contain 3, 4 and 9. In the purple square, there is a number 4 (circled in red) in the right column; that means that the number 4 is eliminated from the two blank spaces in the right column of the upper right square (in orange) and so the number 4 must go in the upper left space of the orange square (marked in blue).
Another way to solve the upper left space of the orange square would have been to simply complete the column – we had already filled in the 8 and the 3 that are marked in blue within that same column; but this just goes to show that there are many different possible ways to solve Sudoku puzzles.
Once we’ve filled in those latest numbers, what does that leave us with? Let’s look at the figure below.
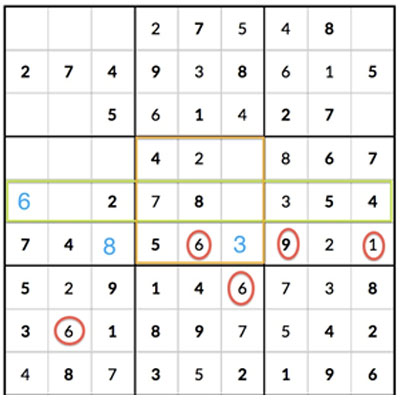
First, look at the row outlined in green that runs through the middle of the grid.
There are only three empty spaces left in this green row, and we know that the missing numbers are 1, 6 and 9.
The middle square (outlined in orange) contains a 6 (circled in red) so that means that the 6 cannot be in the blank space of the green row that is included in that orange square. Also, there is another 6 (circled in red) in the middle column of the left-middle square – so that means that the only place for the 6 to go is in the far left space of the green row (marked in blue).
Let’s look back to the central (orange) square. There are three empty spaces and we know they must contain some combination of 1, 3 and 9. We see in the right square that there is a 1 and 9 (circled in red) in the bottom row, so the lower right corner of the orange square cannot contain either the 1 or the 9 – so it must contain the 3 (marked in blue).
Now if we look at the row (bottom row of the middle set of squares) that the 3 is in, there is only one empty space remaining, and we know it must be number 8 (marked in blue).
We’re getting closer to solving the puzzle.
If you look at the figure below, we can see that the column outlined in green has only two empty spaces, which must contain 3 and 6.
We know from the 6 in the left-central square (circled in red) and also from the 6 in the top row of the right-center square that the one blank space in the green column (within the left-center square) must contain the number 3.
This means that the one remaining blank space in the green column must, by elimination, contain 6.
Now let’s look at the surrounding area where we just placed these new numbers. The upper-left square (where we just placed the 6, in blue) has four remaining empty spaces that must contain 1, 3, 8 and 9.
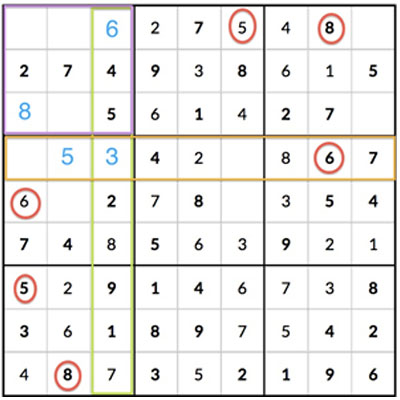
We can see from the 8s in the top row and in the bottom-left square (circled in red) that there is only one possible space in the upper left square (outlined in purple) where the 8 can go. The 8 is now marked in blue.
Now let’s look at the row outlined in orange, where we have placed a number 3 (in blue). This orange row has three remaining empty spaces, which must contain 1, 5 and 9. Two of the spaces can be eliminated as the home for 5, as you can see by the 5s circled in red in the corresponding columns – that means that the 5 must go in the top row, center column of the left-central square (marked in blue).
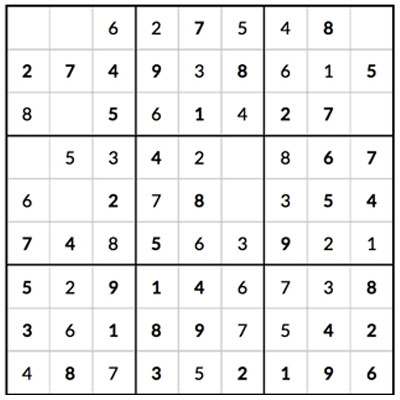
That leaves us with the grid shown at left.
There are only a few empty spaces left to fill in; however, most of the spaces require either a 3 or 9, or a 1 and 9.
At this point, in case you get stuck, you can use the Sudoku.com “Solve” tool to see which numbers are correct.
Sudoku is not supposed to require you to guess, but if you have no other way to solve the puzzle, Sudoku.com allows you to get some help.
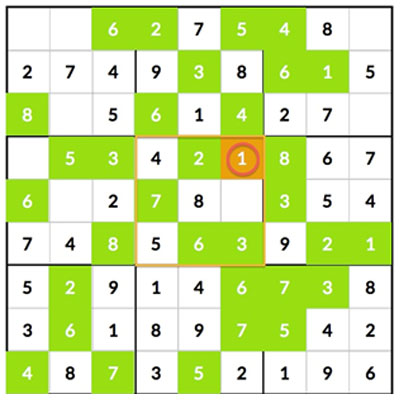
For example, in this figure shown here, in the central square (outlined in orange), we know that there are only two empty spaces left and they must contain 1 and 9, but I cannot figure out which number goes in which space. So I put a 1 in the upper right corner (circled in red) and clicked the word “Solve” below the grid.
After I clicked “Solve,” the number 1’s space was orange – unlike all the other numbers’ spaces which are green. That means that the other numbers (in green) are all correct, but the number 1 (my unlucky guess) is incorrect.
This “Solve” tool shows us that the 1 should actually be a 9.
Now that the central square is solved (with help from the Sudoku.com “Solve” button), the rest of the numbers should fall into place very quickly.
Hopefully this series of articles has been helpful to you in learning how to solve Sudoku puzzles! If you’d like to read the articles from the beginning, here is the rest of the series: